Q: How important is it that insulation be installed with an even depth? Can you average R-values?

A: An even depth of insulation is very important, and No! You cannot average R-values!

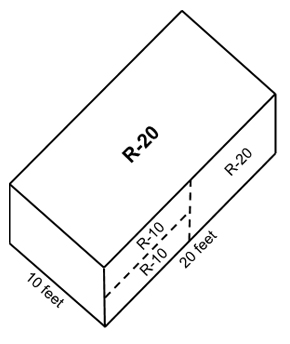
You can add R-values. For instance, in the picture below, you see that R-10 plus R-10 equals R-20. But you cannot average R-values!

R-value has a sister, or cousin, called U-value. U-value Is the inverse of R-value. Or does inverse make it an evil twin? Either way, saying it is an inverse is to say that U-value is equal to 1 divided by the R-value. And conversely, R-value is equal to 1 divided by the U-value. R=1/U and U=1/R.

You can’t add U-values. So what can you do with U-values? You can calculate heat flow, and use that heat flow to find R-values for uneven installations of insulation! While R-value gives you a material’s resistance to heat flow. U-value, the inverse, provides you with a measure of a materials ability to transfer heat.

Just like a car has gas mileage units of miles per gallon, or mpg, R-value has units too. R-value has units of square feet (ft²), times degrees Fahrenheit (F°), times hours (h), divided by British thermal units (btu), or ft² x °F x h / btu. Inversely, remember U = 1/R, U-value has units of British thermal units per hour, per square feet, per degree Fahrenheit, or btu / (h x ft² x °F).

To calculate heat flow for an area of material, like the area of your walls or ceiling, you can use the formula, U-value (U) times Area (A) times the Temperature difference (dT) on both sides of the material, or UxAxdT, or just UAdT. Remember the units? If you look at the formula for calculating heat flow, U btu / (h x ft² x °F) times A ft² times dT °F, you notice that the ft² and °F are in both the numerator and demoninator, and thus cancel leaving us with btu/hr, or heat flow, i.e. how many btu’s of heat will flow in an hour.

Imagine the drawing above represents your attic. Let’s say it’s an unvented attic, and during the day the temperature can get up to 170°F. Inside your home, you keep it a crisp 70°F. The temperature difference, dT, is 100°F. The area, A, is 20 feet by 10 feet, or 200 square feet. It’s a very small house. The R-value is 20, or 20 ft² x °F x h / btu. The U-value then is 1/20, or 0.05 btu/(h x ft² x °F). Then using our formula, UAdT, we have 0.05 btu / (h x ft² x °F) x 200 ft² x 100 °F. Note again that the units of ft² and °F cancel and you are left with btu/h. So in this case, heat would be flowing (always from hot to cold) into our house at a rate of 0.05 x 200 x 100 btu/h, or 1,000 btu per hour.

Btu per hour? That sounds familiar. Like maybe a furnace capacity? But this is a summer time example, so wouldn’t we be using an air conditioner?

Actually, this could just as easily be winter time, with 75°F in the house, and minus 25°F in the attic… because we left Nebraska and went to the mountains skiing… The point being our temperature difference could still be 100°F, and the area and U-value the same as well. All we’ve done is reversed the direction of the flow of heat, and now instead of gaining heat, we are losing heat.

Now, before we go on, I am really oversimplifying just to prove a point. To save energy, I’d try to keep my home at 75° in the summer and 70° in the winter. I'd also vent my attic to make it cooler, approximately 140° F, in the summer. That would reduce my dT and the heat loss or gain. I’d also want to shoot for a much higher R-value in my attic (that would be a lower U-value), which would also reduce heat gain or loss. And of course, I’d probably like to have a home with more than 200 square feet. But as an example this will show the importance of even insulation, and why you can”t average R-value. So back to our small attic.

Let’s imagine we take a fourth of the insulation in our R-20 attic, and move R-10 of that insulation from one half to the other half of the attic. We now have half an attic with an R-10, and the other half with an R-30. Same amount of insulation we had in our original R-20 attic, but no longer evenly spread across the attic. Given our 100°F dT, what’s our heat loss?

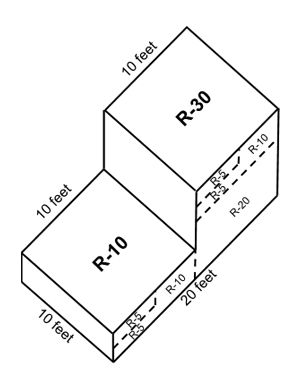
The U-value of R-10 is 1/10 or 0.1, and for an R-30, 1/30, or 0.033. Heat loss on the R-10 side is 0.1 x 100 ft² x 100°F, and on the R-30 side it’s 0.033 x 100 ft² x 100°F. That’s 1,000 btu per hour on the R-10 side and an additional 330 btu per hour on the R-30 side.

But wait! We only had heat flow of 1,000 btu per hour for our entire attic when we had an R-20. Now we have that plus another 330 btu per hour? Yep!

Think about it. In half of the attic you only have half of your original resistance to heat flow, or… twice the heat flow on half the area… the same amount! Plus you have additional heat flow on the R-30 side.

So what’s my actual R-value now?

We now have heat flow of 1,330 btu/h, and that is equal to UAdT, where A is now our total area, dT is still 100°F, and U is the inverse of our new R-value. Or, 1,330 = 200 ft² x 100°F x (U or 1/R). If I move the R to the left of the equal sign, denominator to numerator, and the 1,330 to the right, numerator to denominator, R = 200 x 100 / 1330, or 15. Our R-20 just became an R-15. Another way to look at this, uneven insulation just cost us another 33% (330 btu/h is 33% of 1,000 btu/h).

While an R-value average would tell us we still have an R-20, area weighted U-value tells us we don’t. Once again, NO! You can’t average R-value!

Well, can we say that uneven insulation costs us 25% of the R-value? No! That doesn’t work either.

Let’s take another look, but this time, let’s use an easier way to calculate overall U-values and R-values. It’s called Area Weighted U-value. Formula wise, it looks like this:

UTotal = U1A1 + U2A2 + ….UnAn / ATotal

Once you have calculated UTotal, you simply take the inverse to get your R-value. We can check this with our previous results. U1A1 + U2A2 + ….UnAn in our previous example equals 0.1x100 + 0.033x100 or 10 + 3.3, and ATotal equals 200. UTotal = (10 + 3.3) / 200, or 0.066, and 1/0.066 = R = 15.

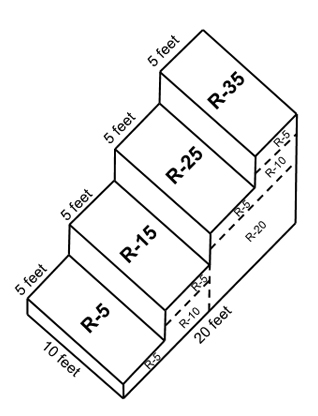
Well yes, and that’s 25% less R-value than we started with. So why can’t we just say that we loose 25% of the R-value if we don’t have even insulation? Because it’s not always 25%! See the little areas of R-5 in the previous drawing? Let’s move some of that R-5 material around and see what happens.

In the drawing of our attic below, with the R-5 material moved around, and using our new formula:

UTotal = U1A1 + U2A2 + ….UnAn / ATotal

U1A1 + U2A2 + ….UnAn is equal to:
1/5 (1/R=U) x 50 + 1/15 x 50 + 1/25 x 50 +1/35 x 50, or
10 + 3.33 + 2 + 1.43, or 16.76.

ATotal is still equal to 200.

UTotal is then equal to 16.76 / 200, or 0.0838

So the R-value of our attic, 1/U, or 1/0.0838 is, R-12.

Whoa! That’s almost half our original R-20 Dude! Now we’ve lost 33% of our original insulation.

Yes, it is. Is having your attic insulation installed evenly important? You bet it is.

Can you average R-value? NO!

Imagine a bunch of dips and swales all across your attic. Or imagine an insulation contractor, or a do it yourselfer, just standing in the attic access, not actually going into the attic, and only blowing insulation as far as their machine will throw the insulation, but piling it up very deep near the access. “Well we put in enough to equal an R-20!” Did you actually get an R-20? No.

This example also applies to flat roofs. Often installers of flat roofs will take the total amount of insulation material used, usually board insulation, and divide the total material by the area that insulation material covers in an attempt to determine the R-value. If we do that with our example, we end up with R-20 every time. But as we’ve shown, the results of a calculation like this are not accurate.

So check your attic, and make sure that you not only have enough insulation, but that you also have a nice even depth of insulation all across your attic. Or, in the case of a flat roof, make sure what you are getting is the result of an area weighted U-value.